Thus, prominent structures in group theory, near-rings, and designs are closely linked. However, historically these areas evolved independently and a general overview is therefore missing.
In the previous years, the relevant topics have been studied at Tainan (Taiwan), Hamburg, and Linz from different points of view. In this project (a joint project with the Taiwanese National Science Council), the respective research groups will cooperate closely. A computer algebra system (``SONATA'') based on GAP and developed at Linz will provide means to study examples of the particular mathematical objects on a computer.
At Linz, we plan to investigate the connections between near-rings, Frobenius groups and designs. Our goal is to reduce the properties of these seemingly distinct algebraic structures to one underlying concept, namely that of Frobenius groups, or, equivalently, to that of fixed-point-free automorphism groups.
This new approach is not only meant to put the results of the respective research areas into relation. It will also provide us with a setting to efficiently attack concrete open problems in the particular fields (Section 2.2):
- determining classes of fixed-point-free automorphism groups on non-abelian groups, and providing construction methods for Frobenius groups of given isomorphism type;
- finding the relation between
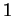 -primitive and planar near-rings;
-primitive and planar near-rings;
- describing the automorphism groups of designs from planar near-rings;
- investigating polynomial and compatible functions on semi-direct products of groups, in particular, Frobenius groups;
- characterizing the
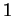 -affine complete Frobenius groups.
-affine complete Frobenius groups.