2.1.1 An overview of the connections between near-rings and Frobenius groups

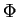We give a rough sketch of the objects we are concerned with and of their interdependencies. Planar near-rings have been used to obtain certain BIB-designs [Fer70]. Finite planar near-rings can be constructed from an additive group $G$ and a group $\Phi$ of automorphisms of $G$ which act fixed-point-freely on $G$ (see [AC68,Cla92,Pil83]). This fact links planar near-rings and Frobenius groups. 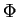Each Frobenius group is a semi-direct product of a group $G$, the Frobenius kernel, which is normal, and a fixed-point-free automorphism group $\Phi$, the Frobenius complement [Hup67].

Fixed-point-free automorphism groups also occur in the characterization of finite simple zero-symmetric near-rings with identity. Such a near-ring is either a ring or isomorphic to a centralizer near-ring,

$$(\mathbf{M}_\Phi(G),+,\circ) = \{ f\colon G\to G\ \vert\ f\circ \varphi=\varphi\circ f\ \textnormal{for all } \varphi\in\Phi\},$$

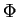where $\Phi$ is a fixed-point-free automorphism group on the group $(G,+)$.

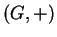We plan to investigate the connections between near-rings, Frobenius groups and designs. One goal is to reduce the properties of seemingly distinct algebraic structures to one underlying concept, namely that of Frobenius groups/fixed-point-free automorphism groups. This approach is meant to bridge the gaps between classical topics which were investigated independently in the past.